Dimensions in Physics
In physics, dimensions refer to the fundamental aspects or parameters that characterize different aspects of the physical universe. These dimensions are used to describe and measure quantities such as length, mass, time, and electric charge. The choice of fundamental dimensions is somewhat arbitrary and depends on the system of units being used.
A derived physical quantity can be expressed in terms of some combination of seven basic or fundamental quantities. For convenience, the basic quantities are represented by symbols as ‘L’ for length, ‘M’ for mass, ‘T’ for time, ‘K’ For temperature, ‘I’ for current, ‘C’ for luminous intensity and ‘mol’ for amount of mass.
The dimensions of a physical quantity are the powers to which the concerned fundamental units must be raised in order to obtain the unit of the given physical quantity.
When we represent any derived quantity with appropriate powers of symbols of the fundamental quantities, then such an expression is called dimensional formula. This dimensional formula is expressed by square bracket and no comma is written in between any of the symbols.
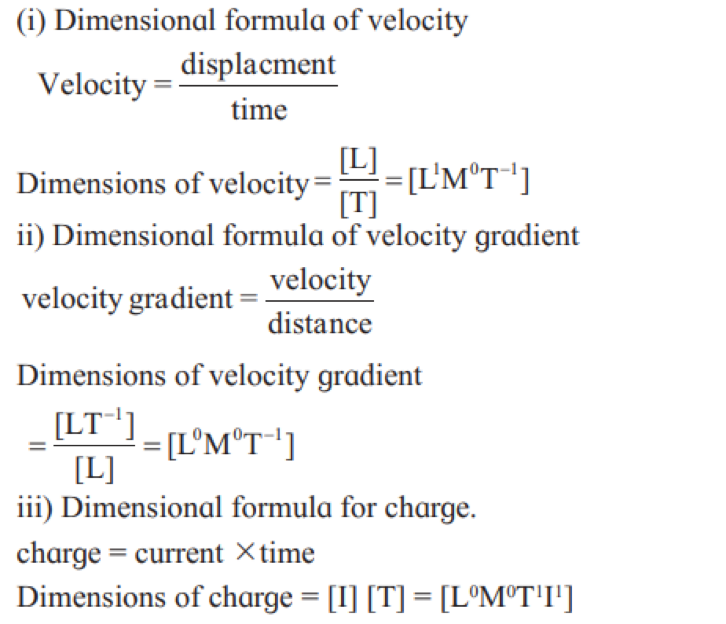
The following table gives the dimensions of various physical quantities commonly used in mechanics.

Limitations of Dimensional Analysis:
1) The value of dimensionless constant can be obtained with the help of experiments only.
2) Dimensional analysis can not be used to derive relations involving trigonometric, exponential, and logarithmic functions as these quantities are dimensionless.
3) This method is not useful if constant of proportionality is not a dimensionless quantity.
Illustration :
Gravitational force between two point masses is directly proportional to product of the two masses and inversely proportional to square of the distance between the two.
The constant of proportionality ‘G’ is NOT dimensionless. Thus earlier method will not work.
4)If the correct equation contains some more terms of the same dimension, it is not possible to know about their presence using dimensional equation. For example, with
standard symbols, the equation S = at2 is dimensionally correct. However, the complete equation is S = ut + at2.