Maxwell’s equations stand as the foundation of electromagnetic theory, providing a comprehensive framework for understanding the fundamental principles governing the behaviour of electric and magnetic fields. Formulated by the Scottish physicist James Clerk Maxwell in the mid-19th century, these equations elegantly describe the interplay between electric charges and currents, shedding light on the nature of electromagnetic waves and the propagation of light. Maxwell’s groundbreaking work has had profound implications for various fields, from telecommunications to modern physics.
Overview of Maxwell's Equations:

Maxwell’s equations consist of four interrelated partial differential equations, encapsulating the intricate relationship between electric and magnetic fields in a given region of space. The equations can be written in both integral and differential forms, offering flexibility in their application to different scenarios. The four equations are:
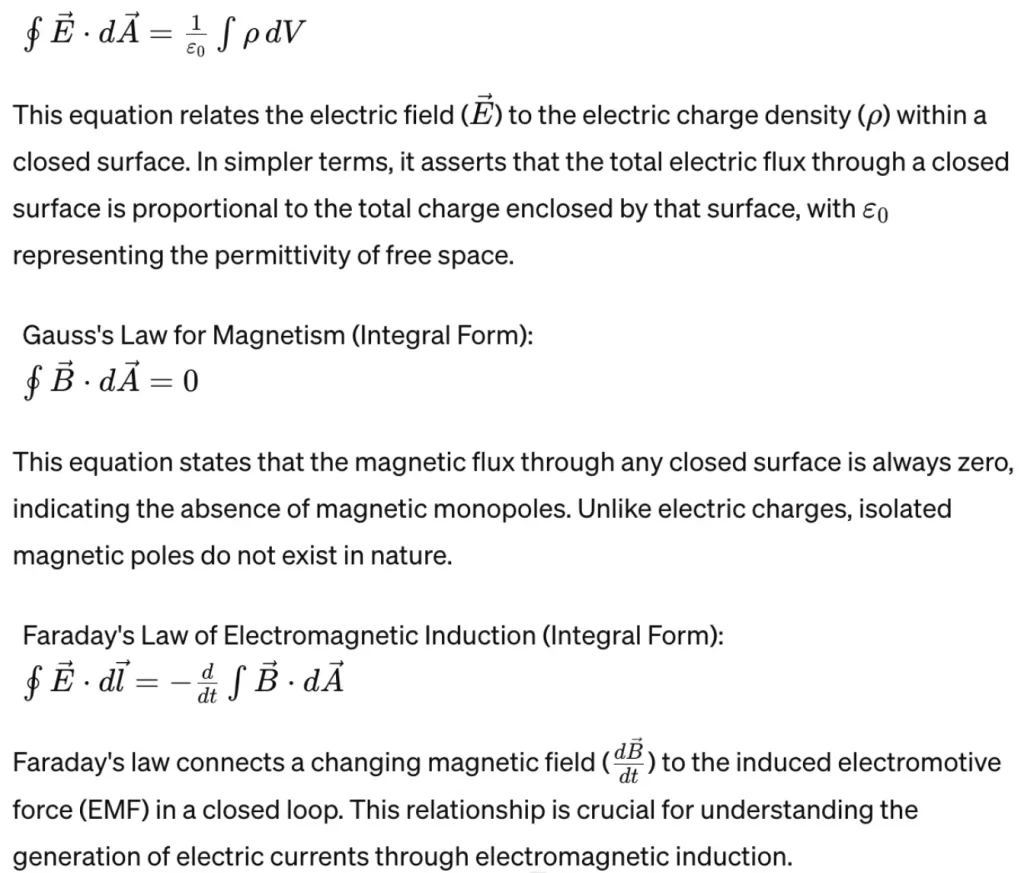
Gauss’s Law for Electricity (Integral Form):

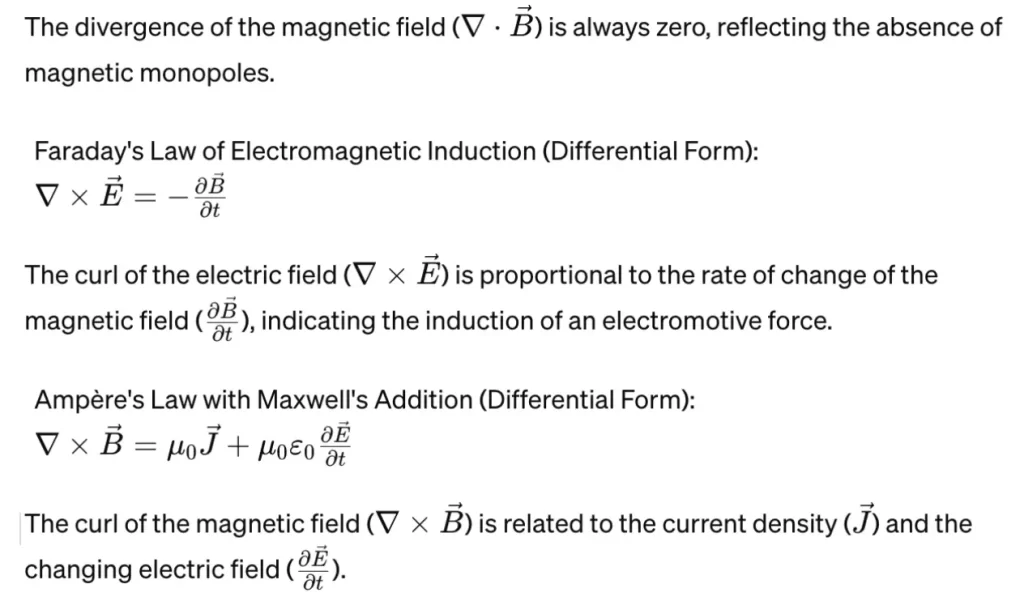
Significance and Applications:
Maxwell’s equations have proven to be invaluable in various scientific and technological fields. Their application extends across:
- Electromagnetic Waves: Maxwell’s equations predict the existence of electromagnetic waves, providing a theoretical foundation for understanding the behaviour of light and other forms of electromagnetic radiation.
- Telecommunications: The equations form the basis for the design and operation of antennas, transmission lines, and communication devices, enabling the development of modern communication systems.
- Electromagnetic Compatibility (EMC): Understanding and applying Maxwell’s equations is crucial for ensuring that electronic devices operate without interference in a shared electromagnetic environment.
- Medical Imaging: Techniques such as magnetic resonance imaging (MRI) and certain methods in medical diagnostics rely on the principles of electromagnetic theory, incorporating Maxwell’s equations into their underlying physics.
- Electromagnetic Propagation: Maxwell’s equations are essential for predicting and controlling the propagation of electromagnetic waves in different media, including the study of reflection, refraction, and diffraction.
Conclusion:
Maxwell’s equations stand as a monumental achievement in the realm of physics, providing a unified framework for understanding the intricate interplay between electric and magnetic fields. Their enduring significance is evident in their widespread application across scientific, technological, and engineering disciplines. As we continue to delve into the complexities of the electromagnetic spectrum, Maxwell’s equations remain an indispensable tool for unraveling the mysteries of the universe and advancing technological innovation.